Polygons CBSE Class 8

What is Polygon?

a plane figure with at least three straight sides and angles, and typically five or more.

Example of Polygons:

Triangle, quadrilateral, pentagon, hexagon, heptagon, octagon, nonagon, decagon according as it contains 3,4,5,6,7,8,9,10 sides respectively.

Different types of polygons:

Concave Polygon: A polygon which has at least one angle is greater than 180° is called concave polygon.

Convex Polygon: A polygon in which all the angles are less than 180° is called convex polygon.

Regular Polygon: A polygon in which all sides and all angles are equal is called regular polygon. Example: equilateral triangle, square.

Irregular Polygon: A polygon in which all sides and all angles are not equal is called irregular polygon. Example: rectangle, rhombus.

1. Find the measure of each exterior angle of a regular  (i) triangle  (ii) 4 sides polygon  (iii) Pentagon  (iv) 10 sides polygon   (v) 15 sides polygon.

Answer:

(i) Each exterior angle of a regular triangle ( equilateral triangle) = (360/3)= 120°

(ii) Each exterior angle of a regular polygon of sides 4 = (360/4) = 90°

(iii) Each exterior angle of a regular pentagon = (360/5) = 72°

(iv) Each exterior angle of a regular polygon of sides 10 = (360/10) = 36°

(v) Each exterior angle of a regular polygon of sides 15 = (360/15) = 24°

 

 You may Like

 1. NCERT Solutions for Class 8 Maths Chapter-wise PDF Download

 

 2.MCQ Questions for  Class 8 Science with Answers Download PDF Solution Chapter Wise

 3.MCQ Questions for  Class 7 Maths with Answers Download PDF Solution Chapter Wise

 4.MCQ Questions for Class 8 Math Chapter 3 Understanding Quadrilaterals with Answers

 

2. Is it possible to have a regular polygon each of whose exterior angles is (i) 60°  (ii) 55°?

Answer:

(i) Let the number of sides of given polygon be n

Therefore, each of its exterior angle = (360/n)°

Now, (360/n) = 60

→ n= 360/60

→ n= 6 (which is a whole number)

So, it is possible to have a regular polygon each of whose exterior angles is 60°

(ii)  Let the number of sides of given polygon be n

Therefore, each of its exterior angle = (360/n)°

Now, (360/n) = 55

→ n = (360/55)

→ n = 72/11 (which is not a whole number)

So, it is not possible to have a regular polygon each of whose exterior angles is 55°

3. Find the measure of each interior angle of a regular polygon having    (i) 10 sides  (ii) 15 sides  (iii) 8 sides.

Answer:

(i) Sum of all exterior angles = 360°

Each exterior angle = (360/10) = 36°

Therefore, each interior angle = (180-36) = 144°

(ii) Sum of all exterior angles = 360°

Each exterior angle = (360/15) = 24°

Therefore, each interior angle = (180-24) = 156°

(iii) Sum of all exterior angles = 360°

Each exterior angle = (360/8) = 45°

Therefore, each interior angle = (180-45) = 135°

(4) Is it possible to have a regular polygon each of whose interior angles is 100°?

Answer:

Each interior angle = 100°

Each exterior angle = (180-100) = 80°

Sum of all exterior angles = 360°

Therefore, Number of sides = (360/80) = (9/2)

(which is not a whole number)

It is not possible.

5. What is the sum of all interior angles of a regular  (i) pentagon  (ii) hexagon  (iii) heptagon?

Answer:

(i) Sum of all interior angles of a regular pentagon = (2  x  5  - 4) right angles

= 6 right angles

(ii) Sum of all interior angles of a regular hexagon

= (2  x  6  - 4) right angles

= 8 right angles

(iii) Sum of all interior angles of a regular heptagon

 = (2  x  7  - 4) right angles

= 10 right angles

6. What is the number of diagonals in a  (i) pentagon  (ii) hexagon  (iii) polygon of 12 sides?

Answer:

(i) Number of diagonals of a polygon of n sides = [n(n-3)/2]

Therefore, number of diagonals of a pentagon = [5(5-3)/2] = 5

(ii) number of diagonals of a pentagon = [6(6-3)/2] = 9

(iii) number of diagonals of a pentagon

 = [12(12-3)/2] = 54

7. Find the number of sides of a regular polygon whose each exterior angles measures   (i) 40°  (ii) 60°  (iii) 90°

Answer:

(i) Sum of all exterior angles of a regular polygon = 360°

Each exterior angle = 40°

Therefore, number of exterior angles = (360/40) = 9

Number of sides of that regular polygon = 9

(ii) Sum of all exterior angles of a regular polygon = 360°

Each exterior angle = 60°

Therefore, number of exterior angles = (360/60) = 6

Number of sides of that regular polygon = 6

(iii) Sum of all exterior angles of a regular polygon = 360°

Each exterior angle = 90°

Therefore, number of exterior angles = (360/90) = 4

Number of sides of that regular polygon = 4

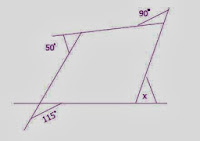
8. In the given figure find the measure ‘x’.

Answer: Sum of all exterior angles of a polygon = 360°

Therefore, 50+115+90+x = 360

               → x = 105°

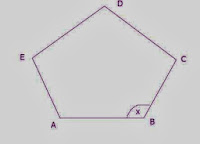
9. Find the angle measure ‘x’ in the given figure.

Answer: Sum of all interior angles of a polygon

 = (2  x  5   -  4) right angles

= 6 right angles

= 6  x  90°

Since this is a regular pentagon

Therefore, x = (6  x  90°)/ /5

               → x = 108°

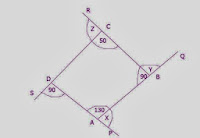
10. Find the value of (x+y+z)

Answer:

Exterior angle + interior adjacent angle = 180°

X = (180 – 130) = 50°

Y = (180 – 90 ) = 90°

Z = (180 – 50) = 130°

(x+y+z) = 50+90+130 = 270°

Some Important Formulas of Polygon:

(i) Sum of all exterior angles = 360°

(ii) Each exterior angle = (360/n)° [n = number of sides]

(iii) Each interior angle = 180° - (each exterior angle)

(iv) Sum of all interior angles = (2n - 4) right angles

(v) Number of diagonals in a polygon of n sides = n(n-3)/2   

7J72I6DH

More Important Searches For You
CBSE Factorisation Mathematics Class VIII(8TH)

Cell: Structure and Function Class VIII CBSE 

CBSE Factorisation Mathematics Class VIII(8TH)

Friction CBSE Class VIII

Probability CBSE Maths

Area of Trapezium, CBSE

Visit My Site Regularly For More New Update on CBSE NCERT CLASS